Spherical Venn Diagrams with Involutory Isometries

Frank Ruskey, Department of Computer Science, University of Victoria, Canada.
Mark Weston, Department of Computer Science, University of Victoria, Canada.

Abstract:

Let f be an isometry of the sphere which is an involution (that is, f-1 = f). For any $n \ge 1$ we prove that there is an $n$-curve Venn diagram on the sphere whose isometry group is generated by f and which has the property that f(C) = C, where C is a curve of the diagram. One of the constructions uses a new chain decomposition of the Boolean lattice, a decomposition which is based on the well-known decomposition of N. G. de Bruijn, C. A. van Ebbenhorst Tengbergen, and D. R. Kruyswijk.

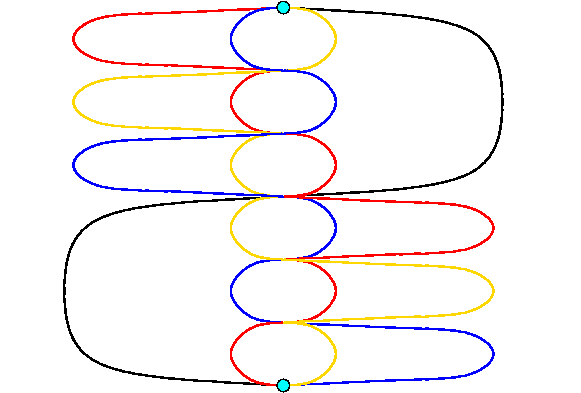
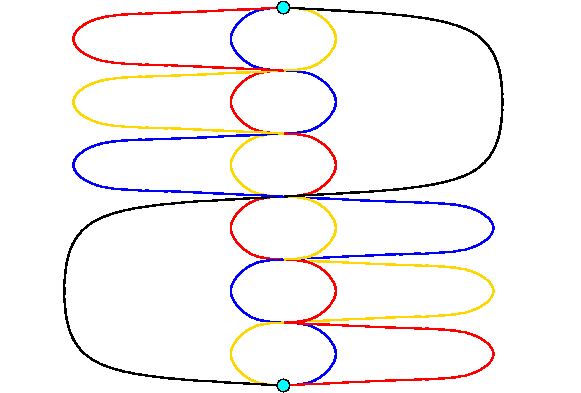
Polar symmetric 4-Venn diagrams

The cyan vertices are identified. On the left, reflect about the center of the sphere. On the right, reflect about the axis through the cyan vertex. These examples can be extended to any n.